
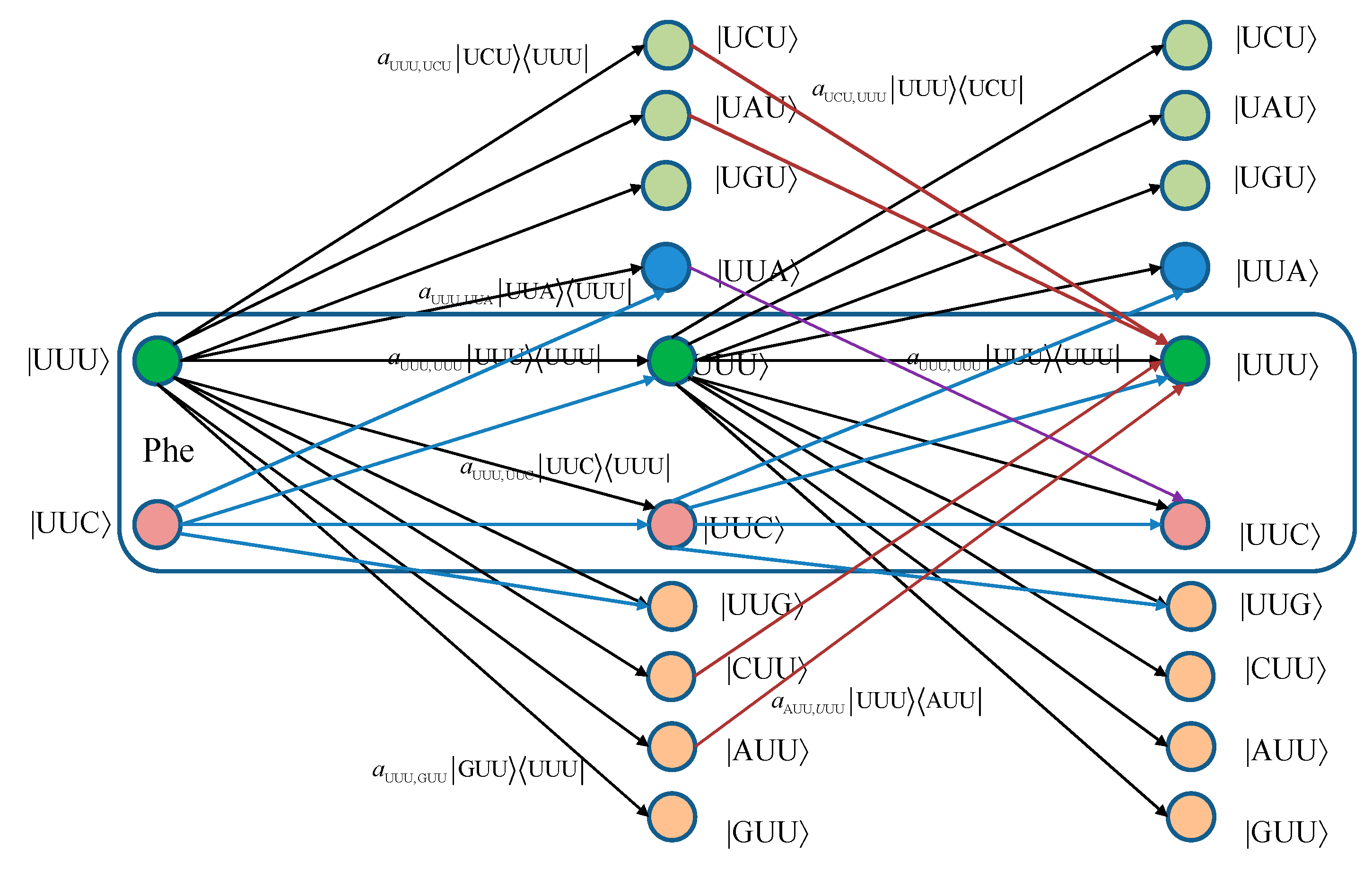
From the definition of stabilizer codes we know that s | i ¯ 〉 = | i ¯ 〉, ∀ s ∈ S. By multiplying both sides by s a †, we obtain s = s a † s b. On the other hand, if there exists an s ∈ S with wt( s) < D, we can find another s a ∈ S so that s as = s b ∈ S.
#Quantum error channels online code#
Because the C q code has a distance D it is clear that wt ( E 2 † E 1 ) < D. Since any correctable error satisfies 〈 i ¯ | E | j ¯ 〉 = C E δ i ¯ j ¯, we obtain 〈 i ¯ | E 2 † E 1 | j ¯ 〉 = C 12 δ i ¯ j ¯. By multiplying by E 2 † from the left we obtain E 2 † E 1 | i ¯ 〉 = | i ¯ 〉, which indicates that the error E 2 † E 1 ∈ S. If the code C q is a degenerate code of distance D, there will exist two correctable errors E 1, E 2 such that their action on a CB codeword is the same: E 1 | i ¯ 〉 = E 2 | i ¯ 〉. The quantum code C q of distance D is a degenerate code if and only if its stabilizer S contains an element with weight less than D (excluding the identity element). The following theorem can be used to determine if a given quantum code C q of quantum distance D is degenerate or not. If we are interested in simultaneously detecting d errors and correcting t errors, the distance of the code must be D ≥ d + t + 1. Since we are interested only in detection of errors we can set E p = I to obtain wt ( E p † E q ) = wt ( E q ) = d. If we are only interested in detecting errors but not correcting them, the error detection capability d is related to the distance D by D ≥ d + 1. Namely, since E = E p † E q the weight of error operator E will be wt ( E p † E q ) = 2 t. Similarly to classical codes, we can relate the distance D to the error correction capability t as follows: D ≥ 2 t + 1. In other words, the distance of QECC is the weight of the smallest weight D of error E that cannot be detected by the code. (7.44), and there exists at least one error of weight D to violate it. We say that a QECC has a distance D if all errors of weight less than D satisfy Eq.


 0 kommentar(er)
0 kommentar(er)
